With about 4500 km separating Rosetta from comet 67P/C-G, the spacecraft will conduct the last of four FAT – ‘Far Approach Trajectory’ – orbit correction manoeuvres later today.
The manoeuvre aims to further reduce Rosetta’s speed with respect to the comet by about 4.82 m/s, such that Rosetta ends the day with a relative speed of 3.5m/s at a distance of 3500 km.
The four FAT burns have been carried out weekly this month, and all have proceeded nominally; the ‘Close Approach Trajectory’ (CAT; pre-insertion and insertion) burns will follow on 3 and 6 August, which will provide further changes in velocity of about 3 and 1 m/s, respectively.
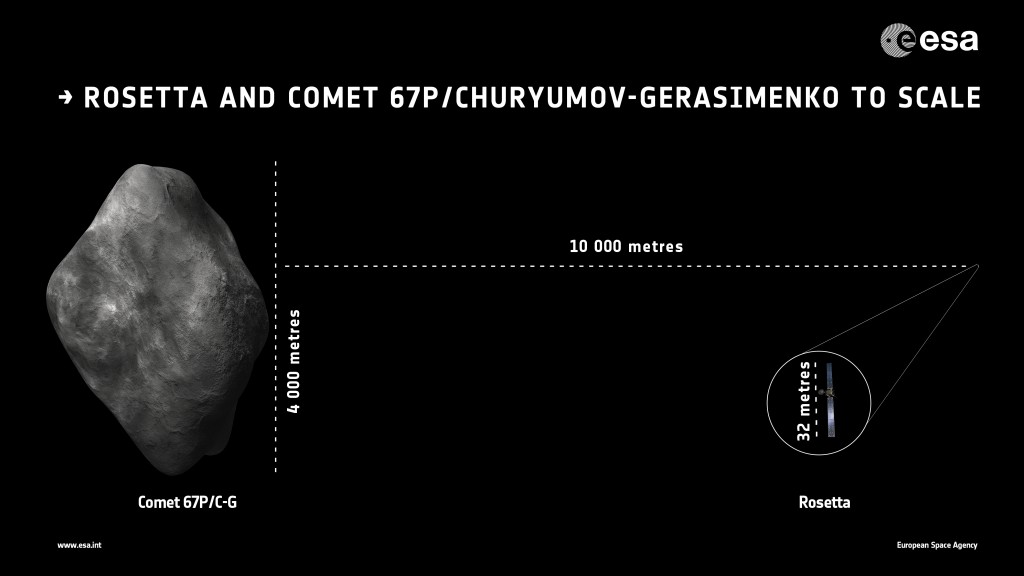
Rosetta and comet 67P/C-G to scale, assuming Rosetta is orbiting at a distance of 10 km, and the comet is about 4 km wide. Credits: ESA.
“Today’s burn starts at 10:38 UTC [12:38 CEST] on board, and is planned to last 16 minutes and 35 seconds,” says Sylvain Lodiot, Rosetta Spacecraft Operations Manager. “All systems on the spacecraft are performing well and the entire team is counting down now the kilometres separating Rosetta from its destination.”
The one-way light time today is 22 mins:26 secs.
Brightest beacons
In the week to 20 July, telecommunications with Rosetta was provided by ESA’s 35m deep-space station at New Norcia, Australia, and NASA’s DSN (Deep Space Network) stations at the Goldstone complex in California.
ESA’s Cebreros, Spain, and Malargüe, Argentina, tracking stations are also being used for the highly sophisticated and very accurate ‘delta-DOR’ (delta differential one-way ranging) navigation technique.
Delta-DOR involves using signals from a quasar located in another galaxy to refine and correct location information derived from Rosetta’s radio signals (see ‘Brightest Beacons’).
Are we there yet?
On 6 August, Rosetta will arrive at the comet at a stand-off distance of 100 km and a relative speed of just 1 m/second.
Arrival will mark the start of an intensive period of scientific data gathering to further characterise the comet and determine candidate landing sites for the Philae lander{LINK}.
In the following weeks, Rosetta will lower its orbit, aiming to get as low as just 10 km; many unknowns remain about 67P/C-G, including its mass, gravitational field and outgassing activity, all of which must be determined and understood before Rosetta can move in that close.
Mass is possibly the most uncertain of all the cometary parameters (because the density is extremely uncertain) yet is the single most important parameter to be estimated from the standpoint of early navigation activities at the comet.
The latest science data – including recent images indicating the comet is a complex ‘contact binary‘ – is enabling the mission team to refine models of the comet. Based on the best estimates of the comet size and shape available before the recent images, the mass is estimated at 3.14×10^12 kg. This will surely change as we learn more about this enigmatic object.
The next OSIRIS images are expected tomorrow.
On 23 July, the comet and Rosetta will be some 403.5 million km from Earth.








Discussion: 25 comments
Very exiting to follow. But please show some real pictures of the comet.
Good luck with the very diffecult steps to made in the coming days.
Kind regards,
Luc
Update at 13:19 CEST from the Rosetta Spacecraft Operations Manager Sylvain Lodiot at ESOC:
Manoeuvre started on board at 10:38:13 UTC and provided a delta-v (change in velocity with respect to the comet) of 4.82 m/s. It was planned for 995 seconds (16min:35sec) and finished 8 seconds early.
All looks good!
Excellent news, the work is all but done now, the CAT burns are very minor.
Minor in duration, but every bit as vital if it is to orbit, and not be a slow fly past. It’s precision that will count now. New pictures tomorrow?
Go Rosetta! Can’t wait fof tomorrows weekly image release.
Good luck to the team !
Definitely much more interesting than a soccer world championship and possibly more important …
Where the OCM’s so far strictly meant to reduce the magnitude of the velocity vector (“braking”)?
Or did they also involve minor changes in the direction of the velocity vector (“course-change”)?
Hi J: Both. I’ll dig out a chart…
What would be the distance for a stationary orbit?
Hi Matthias, Could you be a little more detailed? What sort of ‘stationary orbit’ do you mean? Is there such a thing? Cheers!
I assume he means geostationary, i.e. the distance at which Rosetta’s orbit would match the ~12 hour period of the comet. I think I have read that the mass of the comet has not yet been determined so I suppose this can’t be worked out yet!
It is fascinating to watch this exciting part of the mission after such a long journey!
67-P-o-stationary orbit, analogous to geostationary orbit? Orbital period = comet rotation period of 12.4 hr. A spherical body of the comet’s estimated mass of 3.14×10^12 kg (in the post above) would have an orbital radius of ~2.2 km from the centre of the sphere, and an orbital velocity of 0.3 m/s. But the comet is nowhere near spherical, so such a close orbit would not be stable,
Okay, I mean the orbit where Rosetta would move stationary over the same point of the comet (similar to the geostationary orbit of earth).
At lunch break I did the calculation by myself:
r = cubic-root(G*M*(T/2Pi)²)
G = 6.67E-11 m³/(kg s²)
M = 3.14E12 kg
T = 12.4 * 3600 = 44640s
–> r = 2195m
It seems the orbit would be in the comet itself.
Is this right?
Assuming the equivalent of a geostationary obit is meant, taking a mass of 3.14*10^12kg and a rotational period of 12.7 hours I get a semi major axis of ~2.2km. Thats completely impractical, because its roughly the radius of the comet! You would be orbitting grazing the surface – in the highly non uniform gravitational field which invalidates the calculation anyway.
So I dont think a ’67P – stationary’ orbit is feasible, but I may not have it righ!
If you look at Keplers third law, the mass is vastly lower than the earth’s, but the rotational period is ~ half that of the earth. The only way to get back into ‘sync’ is a very low altitude orbit.
ESA please correct me 🙂
A synchronous orbit, or any stable orbit for that matter, does not appear likely. Note that the assumed mass of 3.2E12 kg or thereabouts would imply a mean density of the nucleus in the order of 100 kg/cubic metre. One tenth of the density of water ice.
That would mean the material is extremely fluffy, most of it just empty space. Looking at how scalloped-out the surface appears, the actual enclosed volume may be significantly reduced, so assuming that that density assumption still holds, the actual mass, and thus the gravity, will be even lower.
It will be interesting to find that out. There are so many interesting things to find out here.
For small bodies, there are two types of stable orbits: retrograde equatorial orbits and self-stabilizing terminator orbits. But I doubt that either of these will work with the low mass we have here.
Orbital perturbations are not just induced by the inhomogeneity of the nucleus’ gravity field but also by the Sun’s gravity and solar radiation pressure. Given the diminutive mass of the nucleus, both perturbing forces are of the same order as the gravity of the comet nucleus.
In a nutshell, although simplifying a bit, orbits that are stable at least for a limited period require the perturbations to be significantly lower then the central body gravity. We don’t have that situation here, I shouldn’t think.
Incidentally, I do hope the mass isn’t 3.141592654….*10^12 or the ‘numerologists’ will have a field day 🙂 But of course its loosing mass by the minute. I stress, 🙂
My post re 67-P -stationary orbits crossed with those of others reaching the same conclusion.
I would have thought stationary in this context would mean a negligible relative velocity with the comet. If it is within the small gravitational pull of the comet, this could be a Lagrangian point or just a very slow orbit.
Perhaps the L1 point so it can see the whole sunlit side while mapping
Any ideas how far away L1 of the sun-67P-system is?
The mass difference is tremendous!
Glad to see I hadn’t messed up the calculation. The result implies that the outer edges of the comet are only just bound, and are moving at close to orbital velocity. If Philae were put in an orbit slightly below the stationary one, it would ‘crash’ into a high-point at a relative speed of a few centimetres per second. And if you tried to walk East there you would go into orbit, if you didn’t sink into the snow first….
Estoy muy emocionado con el encuentro que se dará en breve, esto nos acercará más a comprender la naturaleza del universo, pero seguimos muy lejos de la tolerancia entre paises vecinos 😉
I think L1 & L2 are deep *inside* the comet, whilst L3 is deep inside the Sun. I’ve not tried to do L4, (L5 is identical ‘the other side.) but I think they are very far away.
The huge mass difference causes this.
But someone needs to check I got it right.
I think you are right. The Lagrangian points are irrelevant due to the large mass differences. My point was a kind of “hover” for a time, but in the long run it would use more fuel than orbiting the comet at a safe distance.
The only ‘hover’ would be the Lagrange points, but in fact station-keeping fuel is needed there as the dynamics is complex, and for 67P they are inaccessible. Otherwise your only choice is to orbit, or you’ll burn an awful lot of fuel even against 67P’s tiny gravity, over a long period of time. Orbit is the only game in town.
@L. Foekema: you will find increasingly real pictures of the comet at https://sci.esa.int/rosetta/54389-shape-model-of-comet/
A pity this site doesn’t (yet?) allow for comments.
Otherwise I would have added: At the moment it is looking rather like a human foetus! No more real of course than the “face” on Mars, I hasten to add.